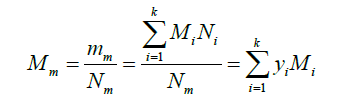Fluid Flow Operation

There are two types of fluid flow in pipelines:

Single-phase flow : gas or liquid

Multi phase flow : a mixture of gas and liquid

1. Single Phase Flow

There are two types of single-phase flow in pipelines : one being designated as streamline flow (sometimes called laminar or viscous flow), and the other as turbulent flow.

1.1 Streamline or Laminar Flow

Streamline or laminar flow is characterized by the gliding of concentric cylindrical layers past one another in orderly fashion.

Velocity of the fluid is at its maximum at the pipe axis and decreases sharply to theoretical zero at the pipe wall.

1.2 Turbulent Flow

In turbulent flow, there is an irregular random motion of fluid particles in directions transverse to the direction of main flow.

The velocity distribution in turbulent flow is more uniform across the pipe diameter than in streamline flow.

1.3 Stages of Flow

At low flow velocity, the streams of liquid flow in straight line. This is Streamline or laminar flow. As the flow rate is gradually increased, these streams will continue to flow in straight lines until a velocity is reached then the streams will waver and commence to break up.

The velocity at which this occurs is called the "Critical Velocity". The type of flow in the critical zone is difficult to determine since it consists of both types.

The nature of the flow in a pipeline, whether streamline or turbulent, depends on the pipe diameter, the density and viscosity of the flowing fluid, and the velocity of the flow. The numerical value of a dimensionless combination of these four variables is known as the Reynolds number and is used to determine the two types of flow.

1.4 Reynolds Number

Reynolds number, NR is a dimensionless number which is of great significance because it can be used to determine the type of flow, either streamline or turbulent, which will occur in any pipeline.

Streamline flow is a characteristic of low gravity, high viscosity liquids flowing at a low rate. Turbulent flow conditions are usually encountered in products pipelines, critical and turbulent flow in crude pipelines except when very viscous liquids are being handled

1.5 Predicting Pressure Loses in Liquid Pipelines

Two empirical formulas are used more often than any others for predicting pressure loss in single-phase systems. These are the Hazen and Williams formula and the Darcy or Fanning equation.

Graphics and flow charts are available for available for prediction of pressure losses in liquid pipeline based on the Hazen and Williams formula .

1.6 Darcy or Fanning Equation

A more accurate method for determining the pressure drop in liquid pipelines is to use the Darcy or Fanning equation.

However, graphs are available for determination of pressure drop in liquid pipelines based on Darcy or Fanning equation.

Expressed in oil industry units, the liquid head loss equation becomes:

1.7 Predicting Pressure Losses in Gas Lines

For estimating pressure drop in short runs of gas piping, Assuming pressure drop through the line is not a significant fraction of the total pressure (i.e. more than 10%).

2. Multi phase Flow

Multi phase vertical flow may be categorized into four different flow configurations or flow regimes, consisting of bubble flow, slug flow, slug-mist transition flow, and mist flow.

Complete sets of pressure traverses for specific flow conditions and oil and gas properties have been published by service companies and others. flow types.

3. Factors Affecting Pressure Losses in Pipelines

∫ Density and viscosity of the fluid

∫ Flowrate or fluid velocity

∫ Pipeline size

∫ Pipeline length

∫ Pipe roughness

∫ Flow restrictions such as valves, chokes and orifices

∫ Pipe fittings and pipeline turns

∫ Changes in elevation

4. Head Loss in Valves and Fittings

When calculating pressure drop inside flow stations, gas-oil separation plants and production units where valves and fittings are a major part of the hoop-up, it is most helpful to convert pressure drops through these fittings to equivalent length of design size piping. Charts are available for this purpose.